We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

TEXT
Unlock Solution Steps
Sign in to
Symbolab
Get full access to all Solution Steps for any math problem
By continuing, you agree to our Terms of Use
and have read our Privacy Policy

Study Guides > College Algebra

Equations of Hyperbolas

Learning Objectives

  • Derive an equation for a hyperbola centered at the origin
  • Write an equation for a hyperbola centered at the origin
  • Solve an applied problem involving hyperbolas
In analytic geometry, a hyperbola is a conic section formed by intersecting a right circular cone with a plane at an angle such that both halves of the cone are intersected. This intersection produces two separate unbounded curves that are mirror images of each other.
A hyperbola
Like the ellipse, the hyperbola can also be defined as a set of points in the coordinate plane. A hyperbola is the set of all points [latex]\left(x,y\right)[/latex] in a plane such that the difference of the distances between [latex]\left(x,y\right)[/latex] and the foci is a positive constant. Notice that the definition of a hyperbola is very similar to that of an ellipse. The distinction is that the hyperbola is defined in terms of the difference of two distances, whereas the ellipse is defined in terms of the sum of two distances. As with the ellipse, every hyperbola has two axes of symmetry. The transverse axis is a line segment that passes through the center of the hyperbola and has vertices as its endpoints. The foci lie on the line that contains the transverse axis. The conjugate axis is perpendicular to the transverse axis and has the co-vertices as its endpoints. The center of a hyperbola is the midpoint of both the transverse and conjugate axes, where they intersect. Every hyperbola also has two asymptotes that pass through its center. 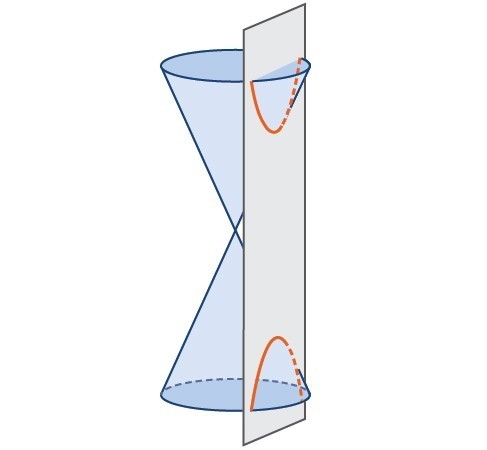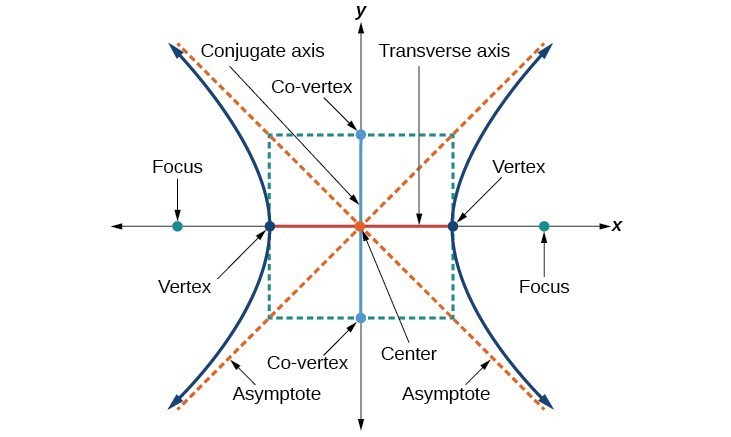As a hyperbola recedes from the center, its branches approach these asymptotes. The central rectangle of the hyperbola is centered at the origin with sides that pass through each vertex and co-vertex; it is a useful tool for graphing the hyperbola and its asymptotes. To sketch the asymptotes of the hyperbola, simply sketch and extend the diagonals of the central rectangle.
Key features of the hyperbola
In this section, we will limit our discussion to hyperbolas that are positioned vertically or horizontally in the coordinate plane; the axes will either lie on or be parallel to the x- and y-axes. We will consider two cases: those that are centered at the origin, and those that are centered at a point other than the origin.

Deriving the Equation of a Hyperbola Centered at the Origin

Let [latex]\left(-c,0\right)[/latex] and [latex]\left(c,0\right)[/latex] be the foci of a hyperbola centered at the origin. The hyperbola is the set of all points [latex]\left(x,y\right)[/latex] such that the difference of the distances from [latex]\left(x,y\right)[/latex] to the foci is constant. If [latex]\left(a,0\right)[/latex] is a vertex of the hyperbola, the distance from [latex]\left(-c,0\right)[/latex] to [latex]\left(a,0\right)[/latex] is [latex]a-\left(-c\right)=a+c[/latex]. The distance from [latex]\left(c,0\right)[/latex] to [latex]\left(a,0\right)[/latex] is [latex]c-a[/latex]. The sum of the distances from the foci to the vertex is

[latex]\left(a+c\right)-\left(c-a\right)=2a[/latex]

If [latex]\left(x,y\right)[/latex] is a point on the hyperbola, we can define the following variables:

[latex]\begin{array}{l}{d}_{2}=\text{the distance from }\left(-c,0\right)\text{ to }\left(x,y\right)\\ {d}_{1}=\text{the distance from }\left(c,0\right)\text{ to }\left(x,y\right)\end{array}[/latex]

By definition of a hyperbola, [latex]{d}_{2}-{d}_{1}[/latex] is constant for any point [latex]\left(x,y\right)[/latex] on the hyperbola. We know that the difference of these distances is [latex]2a[/latex] for the vertex [latex]\left(a,0\right)[/latex]. It follows that [latex]{d}_{2}-{d}_{1}=2a[/latex] for any point on the hyperbola. As with the derivation of the equation of an ellipse, we will begin by applying the distance formula. The rest of the derivation is algebraic. Compare this derivation with the one from the previous section for ellipses.

[latex]\begin{array}{ll}\text{ }{d}_{2}-{d}_{1}=\sqrt{{\left(x-\left(-c\right)\right)}^{2}+{\left(y - 0\right)}^{2}}-\sqrt{{\left(x-c\right)}^{2}+{\left(y - 0\right)}^{2}}=2a\hfill & \text{Distance Formula}\hfill \\ \sqrt{{\left(x+c\right)}^{2}+{y}^{2}}-\sqrt{{\left(x-c\right)}^{2}+{y}^{2}}=2a\hfill & \text{Simplify expressions}\text{.}\hfill \\ \text{ }\sqrt{{\left(x+c\right)}^{2}+{y}^{2}}=2a+\sqrt{{\left(x-c\right)}^{2}+{y}^{2}}\hfill & \text{Move radical to opposite side}\text{.}\hfill \\ \text{ }{\left(x+c\right)}^{2}+{y}^{2}={\left(2a+\sqrt{{\left(x-c\right)}^{2}+{y}^{2}}\right)}^{2}\hfill & \text{Square both sides}\text{.}\hfill \\ \text{ }{x}^{2}+2cx+{c}^{2}+{y}^{2}=4{a}^{2}+4a\sqrt{{\left(x-c\right)}^{2}+{y}^{2}}+{\left(x-c\right)}^{2}+{y}^{2}\hfill & \text{Expand the squares}\text{.}\hfill \\ \text{ }{x}^{2}+2cx+{c}^{2}+{y}^{2}=4{a}^{2}+4a\sqrt{{\left(x-c\right)}^{2}+{y}^{2}}+{x}^{2}-2cx+{c}^{2}+{y}^{2}\hfill & \text{Expand remaining square}\text{.}\hfill \\ \text{ }2cx=4{a}^{2}+4a\sqrt{{\left(x-c\right)}^{2}+{y}^{2}}-2cx\hfill & \text{Combine like terms}\text{.}\hfill \\ \text{ }4cx - 4{a}^{2}=4a\sqrt{{\left(x-c\right)}^{2}+{y}^{2}}\hfill & \text{Isolate the radical}\text{.}\hfill \\ \text{ }cx-{a}^{2}=a\sqrt{{\left(x-c\right)}^{2}+{y}^{2}}\hfill & \text{Divide by 4}\text{.}\hfill \\ \text{ }{\left(cx-{a}^{2}\right)}^{2}={a}^{2}{\left[\sqrt{{\left(x-c\right)}^{2}+{y}^{2}}\right]}^{2}\hfill & \text{Square both sides}\text{.}\hfill \\ \text{ }{c}^{2}{x}^{2}-2{a}^{2}cx+{a}^{4}={a}^{2}\left({x}^{2}-2cx+{c}^{2}+{y}^{2}\right)\hfill & \text{Expand the squares}\text{.}\hfill \\ \text{ }{c}^{2}{x}^{2}-2{a}^{2}cx+{a}^{4}={a}^{2}{x}^{2}-2{a}^{2}cx+{a}^{2}{c}^{2}+{a}^{2}{y}^{2}\hfill & \text{Distribute }{a}^{2}\text{.}\hfill \\ \text{ }{a}^{4}+{c}^{2}{x}^{2}={a}^{2}{x}^{2}+{a}^{2}{c}^{2}+{a}^{2}{y}^{2}\hfill & \text{Combine like terms}\text{.}\hfill \\ \text{ }{c}^{2}{x}^{2}-{a}^{2}{x}^{2}-{a}^{2}{y}^{2}={a}^{2}{c}^{2}-{a}^{4}\hfill & \text{Rearrange terms}\text{.}\hfill \\ \text{ }{x}^{2}\left({c}^{2}-{a}^{2}\right)-{a}^{2}{y}^{2}={a}^{2}\left({c}^{2}-{a}^{2}\right)\hfill & \text{Factor common terms}\text{.}\hfill \\ \text{ }{x}^{2}{b}^{2}-{a}^{2}{y}^{2}={a}^{2}{b}^{2}\hfill & \text{Set }{b}^{2}={c}^{2}-{a}^{2}.\hfill \\ \text{ }\frac{{x}^{2}{b}^{2}}{{a}^{2}{b}^{2}}-\frac{{a}^{2}{y}^{2}}{{a}^{2}{b}^{2}}=\frac{{a}^{2}{b}^{2}}{{a}^{2}{b}^{2}}\hfill & \text{Divide both sides by }{a}^{2}{b}^{2}\hfill \\ \text{ }\frac{{x}^{2}}{{a}^{2}}-\frac{{y}^{2}}{{b}^{2}}=1\hfill & \hfill \end{array}[/latex]

This equation defines a hyperbola centered at the origin with vertices [latex]\left(\pm a,0\right)[/latex] and co-vertices [latex]\left(0\pm b\right)[/latex].

A General Note: Standard Forms of the Equation of a Hyperbola with Center (0,0)

The standard form of the equation of a hyperbola with center [latex]\left(0,0\right)[/latex] and transverse axis on the x-axis is [latex-display]\frac{{x}^{2}}{{a}^{2}}-\frac{{y}^{2}}{{b}^{2}}=1[/latex-display] where
  • the length of the transverse axis is [latex]2a[/latex]
  • the coordinates of the vertices are [latex]\left(\pm a,0\right)[/latex]
  • the length of the conjugate axis is [latex]2b[/latex]
  • the coordinates of the co-vertices are [latex]\left(0,\pm b\right)[/latex]
  • the distance between the foci is [latex]2c[/latex], where [latex]{c}^{2}={a}^{2}+{b}^{2}[/latex]
  • the coordinates of the foci are [latex]\left(\pm c,0\right)[/latex]
  • the equations of the asymptotes are [latex]y=\pm \frac{b}{a}x[/latex]
The standard form of the equation of a hyperbola with center [latex]\left(0,0\right)[/latex] and transverse axis on the y-axis is [latex-display]\frac{{y}^{2}}{{a}^{2}}-\frac{{x}^{2}}{{b}^{2}}=1[/latex-display] where
  • the length of the transverse axis is [latex]2a[/latex]
  • the coordinates of the vertices are [latex]\left(0,\pm a\right)[/latex]
  • the length of the conjugate axis is [latex]2b[/latex]
  • the coordinates of the co-vertices are [latex]\left(\pm b,0\right)[/latex]
  • the distance between the foci is [latex]2c[/latex], where [latex]{c}^{2}={a}^{2}+{b}^{2}[/latex]
  • the coordinates of the foci are [latex]\left(0,\pm c\right)[/latex]
  • the equations of the asymptotes are [latex]y=\pm \frac{a}{b}x[/latex]
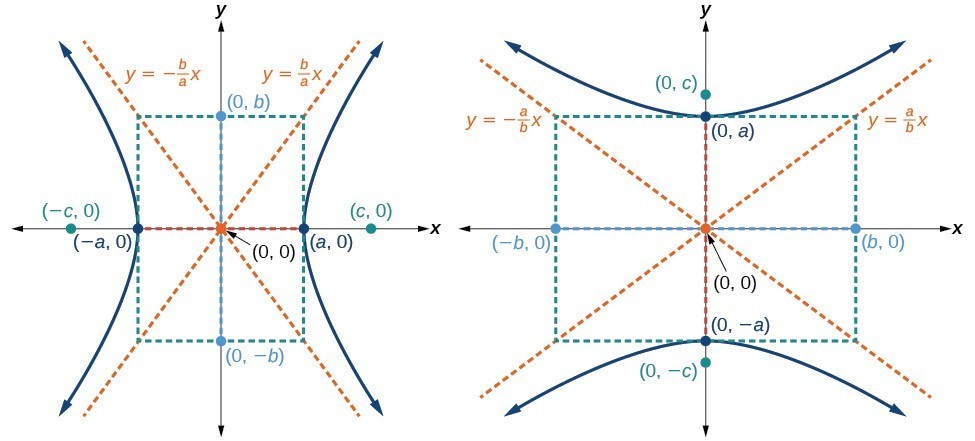Note that the vertices, co-vertices, and foci are related by the equation [latex]{c}^{2}={a}^{2}+{b}^{2}[/latex]. When we are given the equation of a hyperbola, we can use this relationship to identify its vertices and foci.
(a) Horizontal hyperbola with center [latex]\left(0,0\right)[/latex] (b) Vertical hyperbola with center [latex]\left(0,0\right)[/latex]

How To: Given the equation of a hyperbola in standard form, locate its vertices and foci.

  1. Determine whether the transverse axis lies on the x- or y-axis. Notice that [latex]{a}^{2}[/latex] is always under the variable with the positive coefficient. So, if you set the other variable equal to zero, you can easily find the intercepts. In the case where the hyperbola is centered at the origin, the intercepts coincide with the vertices.
a. If the equation has the form [latex]\frac{{x}^{2}}{{a}^{2}}-\frac{{y}^{2}}{{b}^{2}}=1[/latex], then the transverse axis lies on the x-axis. The vertices are located at [latex]\left(\pm a,0\right)[/latex], and the foci are located at [latex]\left(\pm c,0\right)[/latex]. b. If the equation has the form [latex]\frac{{y}^{2}}{{a}^{2}}-\frac{{x}^{2}}{{b}^{2}}=1[/latex], then the transverse axis lies on the y-axis. The vertices are located at [latex]\left(0,\pm a\right)[/latex], and the foci are located at [latex]\left(0,\pm c\right)[/latex].
  1. Solve for [latex]a[/latex] using the equation [latex]a=\sqrt{{a}^{2}}[/latex].
  2. Solve for [latex]c[/latex] using the equation [latex]c=\sqrt{{a}^{2}+{b}^{2}}[/latex].

Example: Locating a Hyperbola’s Vertices and Foci

Identify the vertices and foci of the hyperbola with equation [latex]\frac{{y}^{2}}{49}-\frac{{x}^{2}}{32}=1[/latex].

Answer: The equation has the form [latex]\frac{{y}^{2}}{{a}^{2}}-\frac{{x}^{2}}{{b}^{2}}=1[/latex], so the transverse axis lies on the y-axis. The hyperbola is centered at the origin, so the vertices serve as the y-intercepts of the graph. To find the vertices, set [latex]x=0[/latex], and solve for [latex]y[/latex].

[latex]\begin{array}{l}1=\frac{{y}^{2}}{49}-\frac{{x}^{2}}{32}\hfill \\ 1=\frac{{y}^{2}}{49}-\frac{{0}^{2}}{32}\hfill \\ 1=\frac{{y}^{2}}{49}\hfill \\ {y}^{2}=49\hfill \\ y=\pm \sqrt{49}=\pm 7\hfill \end{array}[/latex]

The foci are located at [latex]\left(0,\pm c\right)[/latex]. Solving for [latex]c[/latex],

[latex]c=\sqrt{{a}^{2}+{b}^{2}}=\sqrt{49+32}=\sqrt{81}=9[/latex]

Therefore, the vertices are located at [latex]\left(0,\pm 7\right)[/latex], and the foci are located at [latex]\left(0,9\right)[/latex].

Try It

Identify the vertices and foci of the hyperbola with equation [latex]\frac{{x}^{2}}{9}-\frac{{y}^{2}}{25}=1[/latex].

Answer: Vertices: [latex]\left(\pm 3,0\right)[/latex]; Foci: [latex]\left(\pm \sqrt{34},0\right)[/latex]

Hyperbolas Not Centered at the Origin

Like the graphs for other equations, the graph of a hyperbola can be translated. If a hyperbola is translated [latex]h[/latex] units horizontally and [latex]k[/latex] units vertically, the center of the hyperbola will be [latex]\left(h,k\right)[/latex]. This translation results in the standard form of the equation we saw previously, with [latex]x[/latex] replaced by [latex]\left(x-h\right)[/latex] and [latex]y[/latex] replaced by [latex]\left(y-k\right)[/latex].

A General Note: Standard Forms of the Equation of a Hyperbola with Center (h, k)

The standard form of the equation of a hyperbola with center [latex]\left(h,k\right)[/latex] and transverse axis parallel to the x-axis is [latex-display]\frac{{\left(x-h\right)}^{2}}{{a}^{2}}-\frac{{\left(y-k\right)}^{2}}{{b}^{2}}=1[/latex-display] where
  • the length of the transverse axis is [latex]2a[/latex]
  • the coordinates of the vertices are [latex]\left(h\pm a,k\right)[/latex]
  • the length of the conjugate axis is [latex]2b[/latex]
  • the coordinates of the co-vertices are [latex]\left(h,k\pm b\right)[/latex]
  • the distance between the foci is [latex]2c[/latex], where [latex]{c}^{2}={a}^{2}+{b}^{2}[/latex]
  • the coordinates of the foci are [latex]\left(h\pm c,k\right)[/latex]
The asymptotes of the hyperbola coincide with the diagonals of the central rectangle. The length of the rectangle is [latex]2a[/latex] and its width is [latex]2b[/latex]. The slopes of the diagonals are [latex]\pm \frac{b}{a}[/latex], and each diagonal passes through the center [latex]\left(h,k\right)[/latex]. Using the point-slope formula, it is simple to show that the equations of the asymptotes are [latex]y=\pm \frac{b}{a}\left(x-h\right)+k[/latex]. The standard form of the equation of a hyperbola with center [latex]\left(h,k\right)[/latex] and transverse axis parallel to the y-axis is [latex-display]\frac{{\left(y-k\right)}^{2}}{{a}^{2}}-\frac{{\left(x-h\right)}^{2}}{{b}^{2}}=1[/latex-display] where
  • the length of the transverse axis is [latex]2a[/latex]
  • the coordinates of the vertices are [latex]\left(h,k\pm a\right)[/latex]
  • the length of the conjugate axis is [latex]2b[/latex]
  • the coordinates of the co-vertices are [latex]\left(h\pm b,k\right)[/latex]
  • the distance between the foci is [latex]2c[/latex], where [latex]{c}^{2}={a}^{2}+{b}^{2}[/latex]
  • the coordinates of the foci are [latex]\left(h,k\pm c\right)[/latex]
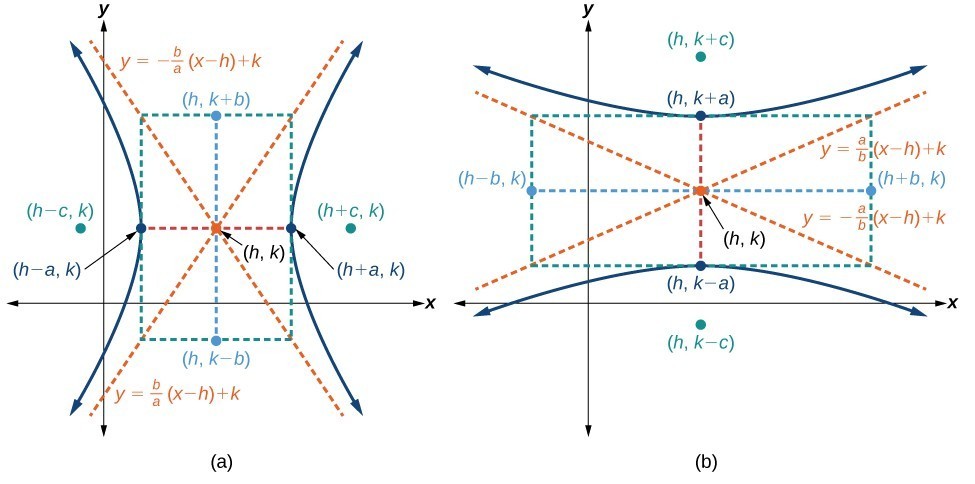Using the reasoning above, the equations of the asymptotes are [latex]y=\pm \frac{a}{b}\left(x-h\right)+k[/latex].
(a) Horizontal hyperbola with center [latex]\left(h,k\right)[/latex] (b) Vertical hyperbola with center [latex]\left(h,k\right)[/latex]
Like hyperbolas centered at the origin, hyperbolas centered at a point [latex]\left(h,k\right)[/latex] have vertices, co-vertices, and foci that are related by the equation [latex]{c}^{2}={a}^{2}+{b}^{2}[/latex]. We can use this relationship along with the midpoint and distance formulas to find the standard equation of a hyperbola when the vertices and foci are given.

How To: Given the vertices and foci of a hyperbola centered at [latex]\left(h,k\right)[/latex], write its equation in standard form.

  1. Determine whether the transverse axis is parallel to the x- or y-axis.
    1. If the y-coordinates of the given vertices and foci are the same, then the transverse axis is parallel to the x-axis. Use the standard form [latex]\frac{{\left(x-h\right)}^{2}}{{a}^{2}}-\frac{{\left(y-k\right)}^{2}}{{b}^{2}}=1[/latex].
    2. If the x-coordinates of the given vertices and foci are the same, then the transverse axis is parallel to the y-axis. Use the standard form [latex]\frac{{\left(y-k\right)}^{2}}{{a}^{2}}-\frac{{\left(x-h\right)}^{2}}{{b}^{2}}=1[/latex].
  2. Identify the center of the hyperbola, [latex]\left(h,k\right)[/latex], using the midpoint formula and the given coordinates for the vertices.
  3. Find [latex]{a}^{2}[/latex] by solving for the length of the transverse axis, [latex]2a[/latex] , which is the distance between the given vertices.
  4. Find [latex]{c}^{2}[/latex] using [latex]h[/latex] and [latex]k[/latex] found in Step 2 along with the given coordinates for the foci.
  5. Solve for [latex]{b}^{2}[/latex] using the equation [latex]{b}^{2}={c}^{2}-{a}^{2}[/latex].
  6. Substitute the values for [latex]h,k,{a}^{2}[/latex], and [latex]{b}^{2}[/latex] into the standard form of the equation determined in Step 1.

Example: Finding the Equation of a Hyperbola Centered at (h, k) Given its Foci and Vertices

What is the standard form equation of the hyperbola that has vertices at [latex]\left(0,-2\right)[/latex] and [latex]\left(6,-2\right)[/latex] and foci at [latex]\left(-2,-2\right)[/latex] and [latex]\left(8,-2\right)?[/latex]

Answer: The y-coordinates of the vertices and foci are the same, so the transverse axis is parallel to the x-axis. Thus, the equation of the hyperbola will have the form

[latex]\frac{{\left(x-h\right)}^{2}}{{a}^{2}}-\frac{{\left(y-k\right)}^{2}}{{b}^{2}}=1[/latex]

First, we identify the center, [latex]\left(h,k\right)[/latex]. The center is halfway between the vertices [latex]\left(0,-2\right)[/latex] and [latex]\left(6,-2\right)[/latex]. Applying the midpoint formula, we have

[latex]\left(h,k\right)=\left(\frac{0+6}{2},\frac{-2+\left(-2\right)}{2}\right)=\left(3,-2\right)[/latex]

Next, we find [latex]{a}^{2}[/latex]. The length of the transverse axis, [latex]2a[/latex], is bounded by the vertices. So, we can find [latex]{a}^{2}[/latex] by finding the distance between the x-coordinates of the vertices.

[latex]\begin{array}{l}2a=|0 - 6|\hfill \\ 2a=6\hfill \\ \text{ }a=3\hfill \\ {a}^{2}=9\hfill \end{array}[/latex]

Now we need to find [latex]{c}^{2}[/latex]. The coordinates of the foci are [latex]\left(h\pm c,k\right)[/latex]. So [latex]\left(h-c,k\right)=\left(-2,-2\right)[/latex] and [latex]\left(h+c,k\right)=\left(8,-2\right)[/latex]. We can use the x-coordinate from either of these points to solve for [latex]c[/latex]. Using the point [latex]\left(8,-2\right)[/latex], and substituting [latex]h=3[/latex],

[latex]\begin{array}{l}h+c=8\hfill \\ 3+c=8\hfill \\ \text{ }c=5\hfill \\ \text{ }{c}^{2}=25\hfill \end{array}[/latex]

Next, solve for [latex]{b}^{2}[/latex] using the equation [latex]{b}^{2}={c}^{2}-{a}^{2}:[/latex]

[latex]\begin{array}{l}{b}^{2}={c}^{2}-{a}^{2}\hfill \\ \text{ }=25 - 9\hfill \\ \text{ }=16\hfill \end{array}[/latex]

Finally, substitute the values found for [latex]h,k,{a}^{2}[/latex], and [latex]{b}^{2}[/latex] into the standard form of the equation.

[latex]\frac{{\left(x - 3\right)}^{2}}{9}-\frac{{\left(y+2\right)}^{2}}{16}=1[/latex]

Try It

What is the standard form equation of the hyperbola that has vertices [latex]\left(1,-2\right)[/latex] and [latex]\left(1,\text{8}\right)[/latex] and foci [latex]\left(1,-10\right)[/latex] and [latex]\left(1,16\right)?[/latex]

Answer: [latex]\frac{{\left(y - 3\right)}^{2}}{25}+\frac{{\left(x - 1\right)}^{2}}{144}=1[/latex]

Solving Applied Problems Involving Hyperbolas

As we discussed at the beginning of this section, hyperbolas have real-world applications in many fields, such as astronomy, physics, engineering, and architecture. The design efficiency of hyperbolic cooling towers is particularly interesting. Cooling towers are used to transfer waste heat to the atmosphere and are often touted for their ability to generate power efficiently. Because of their hyperbolic form, these structures are able to withstand extreme winds while requiring less material than any other forms of their size and strength. For example, a 500-foot tower can be made of a reinforced concrete shell only 6 or 8 inches wide!
description in caption Cooling towers at the Drax power station in North Yorkshire, United Kingdom (credit: Les Haines, Flickr)
The first hyperbolic towers were designed in 1914 and were 35 meters high. Today, the tallest cooling towers are in France, standing a remarkable 170 meters tall. In Example 6 we will use the design layout of a cooling tower to find a hyperbolic equation that models its sides.

Example: Solving Applied Problems Involving Hyperbolas

The design layout of a cooling tower is shown below. The tower stands 179.6 meters tall. The diameter of the top is 72 meters. At their closest, the sides of the tower are 60 meters apart.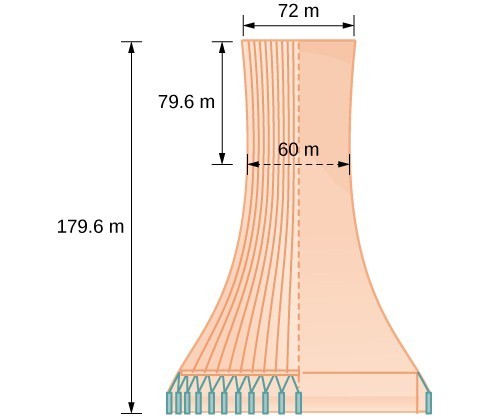
Project design for a natural draft cooling tower
Find the equation of the hyperbola that models the sides of the cooling tower. Assume that the center of the hyperbola—indicated by the intersection of dashed perpendicular lines in the figure—is the origin of the coordinate plane. Round final values to four decimal places.

Answer: We are assuming the center of the tower is at the origin, so we can use the standard form of a horizontal hyperbola centered at the origin: [latex]\frac{{x}^{2}}{{a}^{2}}-\frac{{y}^{2}}{{b}^{2}}=1[/latex], where the branches of the hyperbola form the sides of the cooling tower. We must find the values of [latex]{a}^{2}[/latex] and [latex]{b}^{2}[/latex] to complete the model. First, we find [latex]{a}^{2}[/latex]. Recall that the length of the transverse axis of a hyperbola is [latex]2a[/latex]. This length is represented by the distance where the sides are closest, which is given as [latex]\text{ }65.3\text{ }[/latex] meters. So, [latex]2a=60[/latex]. Therefore, [latex]a=30[/latex] and [latex]{a}^{2}=900[/latex]. To solve for [latex]{b}^{2}[/latex], we need to substitute for [latex]x[/latex] and [latex]y[/latex] in our equation using a known point. To do this, we can use the dimensions of the tower to find some point [latex]\left(x,y\right)[/latex] that lies on the hyperbola. We will use the top right corner of the tower to represent that point. Since the y-axis bisects the tower, our x-value can be represented by the radius of the top, or 36 meters. The y-value is represented by the distance from the origin to the top, which is given as 79.6 meters. Therefore,

[latex]\begin{array}{ll}\frac{{x}^{2}}{{a}^{2}}-\frac{{y}^{2}}{{b}^{2}}=1\hfill & \begin{array}{cccc}& & & \end{array}\text{Standard form of horizontal hyperbola}.\hfill \\ \text{ }{b}^{2}=\frac{{y}^{2}}{\frac{{x}^{2}}{{a}^{2}}-1}\hfill & \begin{array}{cccc}& & & \end{array}\text{Isolate }{b}^{2}\hfill \\ \text{ }=\frac{{\left(79.6\right)}^{2}}{\frac{{\left(36\right)}^{2}}{900}-1}\hfill & \begin{array}{cccc}& & & \end{array}\text{Substitute for }{a}^{2},x,\text{ and }y\hfill \\ \text{ }\approx 14400.3636\hfill & \begin{array}{cccc}& & & \end{array}\text{Round to four decimal places}\hfill \end{array}[/latex]

The sides of the tower can be modeled by the hyperbolic equation

[latex]\frac{{x}^{2}}{900}-\frac{{y}^{2}}{14400.3636 }=1,\text{or}\frac{{x}^{2}}{{30}^{2}}-\frac{{y}^{2}}{{120.0015}^{2} }=1[/latex]

Try It

A design for a cooling tower project is shown below. Find the equation of the hyperbola that models the sides of the cooling tower. Assume that the center of the hyperbola—indicated by the intersection of dashed perpendicular lines in the figure—is the origin of the coordinate plane. Round final values to four decimal places.

Answer: The sides of the tower can be modeled by the hyperbolic equation. [latex]\frac{{x}^{2}}{400}-\frac{{y}^{2}}{3600}=1\text{or }\frac{{x}^{2}}{{20}^{2}}-\frac{{y}^{2}}{{60}^{2}}=1[/latex].

Licenses & Attributions

CC licensed content, Original

CC licensed content, Shared previously

  • College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. Located at: https://openstax.org/books/college-algebra/pages/1-introduction-to-prerequisites. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/[email protected].
  • Question ID 1669. Authored by: WebWork-Rochester, mb Sousa,James. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
  • Question ID 70367. Authored by: Shahbazian,Roy. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.